determine the changing point of temperature curve

[dragon-long]

Hello

I colelcted data using NI 9213

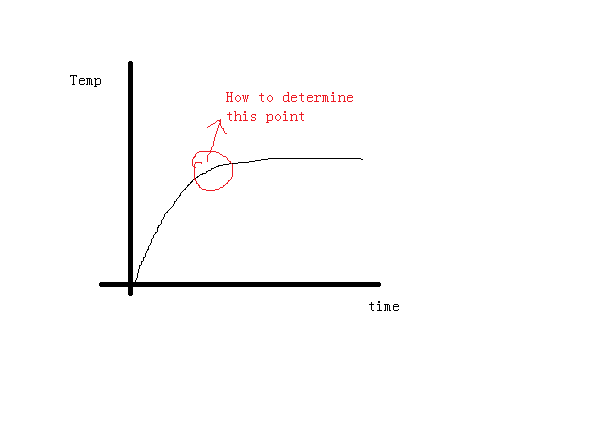
My temperature curve will be like this . What kind of function can I use this changing point in this Temp curve?

I may use derivative of slope, right? The other problem is my curve may not as sooth as what I draw. They may some some bump..

I need to filter the noise??

Thank you for your help

[dragon-long]

My experimental curve will be more like this.

I am not sure how I determine this point.

Also this point is one trigger scource. So I hope I can get it once this point showed up then I need to change my process state.

Thanks

[crossrulz]

Well, let's start off with a simple question.  How do you, looking at the graph, determine this point?  What exactly do you mean by "changing point".

[dragon-long]

I think it is the first point to reach the equilibrium temperature. After this point , T is still increasing . but not a lot , may be only 1 degree C increase. So I cannot say  this T is the maximum Temperature. Also in my actual curve, they have several thresholds, I am not sure how to determine the threshold I want which is close to Equilibrium T.

[johnsold]

The points you have marked in both images are NOT the first time the temperature reached the equilibrium temperature.

You seem to be implying that you get some overshoot.  Is that what is happening?

Do you know what the equilibrium temperature will be before the system settles to equilibrium?

Please post an image of a plot of actual data.

Lynn

[dragon-long]

[dragon-long]

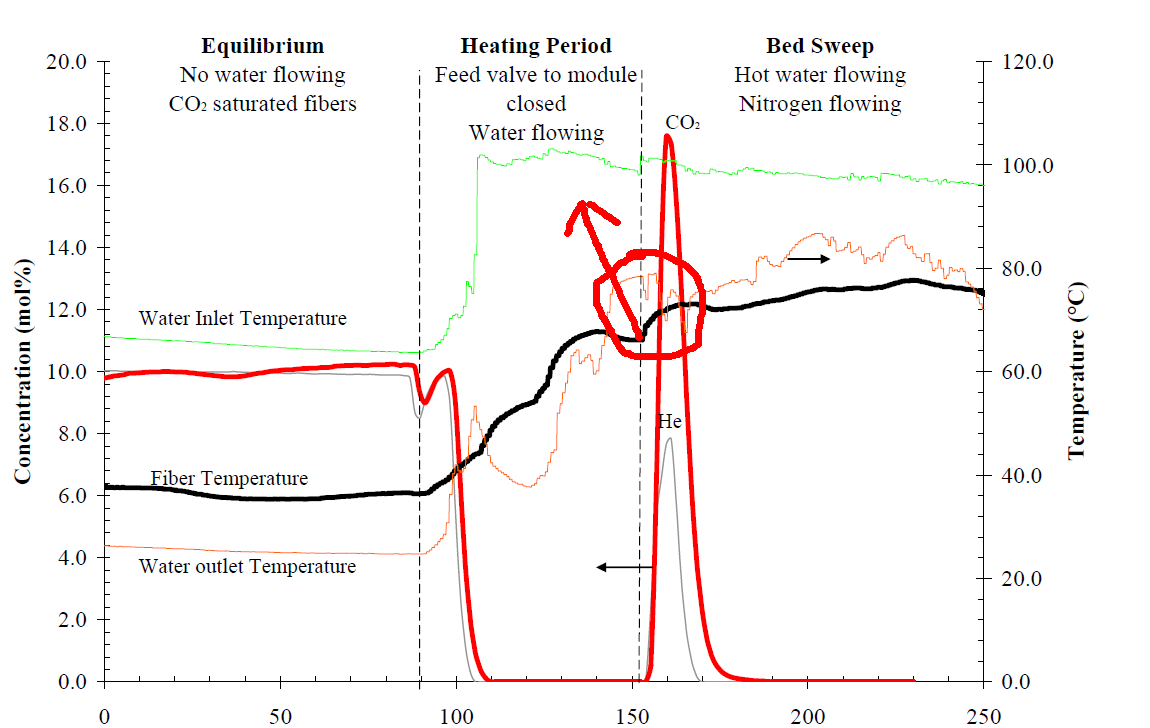
Actual data will be like this

Here we only look at fiber temperature

When Fiber Temperature curve almost look like a plataeu , We immediately move process from heating period to bed sweep stage.

[crossrulz]

I'm kind of thinking performing a moving average and then taking the derivative on the average.  When the derivative is small enough (1 degree/s?), you reached your point.

[johnsold]

That is pretty scary data on which to attempt an automated analysis.

The only thing I see there which might work is to first identify the broad peak which occurs at 135-140 on the horizontal axis then look for an approach to close to zero slope after that.  Because the differentaition process to get the slope is an inherently noisy process, you may need to do some smoothing or qualify the data by requiring the conditions to be met for some period of time before deciding.  Looking at only one data set does not give me enough information to judge the reproducibility or noise levels in your signals.

Do you have knowledge from theory which predicts a mathematical formula for the shape of that curve? Extra knowledge can often be used to make a better decision.

Lynn

[dragon-long]

This may be a good idea. 

Let me think about it